And so we’ve lost our math major

The mathematics major is not one that often gets cut, but that is what happened to us. I admit that I saw it coming. Yes, there will be litigation, law suits etc, but most of that is personnel oriented. The fact is that, for now, our math major is gone; not that we won’t try to bring it back when our current top administrators leave, one way or another.

Yes, tenured faculty were cut. That is where litigation will come in. And yes, there is grief over that…a lot of it.

But that is not what this post will focus on.

For some of my colleagues, there is a profound sense of grief of being a math professor at a university that will no longer offer a math major. For them: a big part of their identity was being a mathematician.

Sadly, that isn’t true of me; I lost my identity of being a mathematician decades ago.

Time and time again, I’d go to a conference, be fired up about what I saw, return to campus…and watched the ideas in my head die a slow death under 11-12 hour teaching loads, committee work, etc. Over time, I started switching to MAA type conferences (Mathfest, for example) and finding joy in teaching “out of area” courses, even actuarial science ones. I sort of became “known” for that.

And yeah, I did research, picking off a problem here and there and did enough to make Full Professor at my institution. But more and more, by research became more shallow and more broad. And frankly I lost the appetite for all of the BS that comes with publication (type setting, figures, dealing with pedantic referees, etc) Yes, I have a paper at the referee’s now and I owe a referee’s report as well.

But..well, when I see what the mathematicians are doing at the R1 universities (e. g. Big Ten);, well, what I do simply does not compare..it never did. I came to accept that decades ago.

This is a long winded way of saying that I suffered no ego bruise from this.

Note: I am not saying this is totally over; if/when we get better leardership I might attempt to bring a math major back (yes, I am the incoming chair of the department).

Moving forward: I think that the math major is on the wane, at least at the non-elite universities.

Just shoot me. Now. (personal…and my screw up…)

The upcoming semester line up:

1. My original schedule called for science/engineering calculus II, “business calculus” I, and numerical analysis. No, my specialty is pure math (topology) but I got roped into teaching this course a few years ago, and because no one complained…guess who is stuck with it now? 🙂 (and yes, as an undergraduate, and once as a part time graduate student, I made C’s in this class)

2. But a part time faculty who taught our actuarial mathematics classes got called away so..I said “if you can’t find anyone else…” and so I got stuck with that class (in lieu of the “business calculus” class)

3. I get an e-mail about the class; I read the Fall 2014 syllabus and so prepare based on that (since it is Fall 2015)..but the topics for that class go “spring, fall, off, fall, spring” instead of “fall/spring”…so I had prepared FOR THE WRONG CLASS and ordered THE WRONG BOOK.

I caught that a week prior to classes starting..hence frantic e-mail to the department chair and secretary….and I’ll have to really work some this weekend.

Fortunately much of the stuff in this topic (“life contingencies”) is like reliability engineering and I’ve had that class. Things like the “survival function” and “bathtub curve” are familiar to me. The mathematics won’t be hard; I’ll have to focus my self study on definitions and notation.

Still, this is more interesting than I’d hoped that it would be.

The positive: I’ll have learned some new mathematics (I always learn something new every time I teach numerical analysis) and new applications of mathematics (the life contingencies …and I’ve already learned a little bit of interest theory by preparing for the class that I thought that I was teaching..)

The university
But our university (6000 students, 5000 undergraduates) is suffering from an enrollment slump for the second year in a row. We are at about 85-88 percent of what would be a “healthy” enrollment. The place is in turmoil; we lost our athletic director, provost (left) and president, the flagship basketball team has hit rock bottom and things are in disarray.

So we have a second “small” class but this time: enrollments are UP in our remedial sections. UP. And many who couldn’t place into our regular calculus sequence have been admitted…by …engineering. Seriously. They are hurting that badly, and when they hurt, WE hurt.

I’ll be shielded from much of that in the classroom because of the classes I am teaching BUT with these changes come “changes in major”; we are going to try to make our major easier to navigate by trying to maximize flexibility by making our “required courses” less prerequisite dependent. It will water down the major somewhat but hopefully make it more likely that we keep a major.

Oh well…this is what I get for taking my Ph. D. in pure mathematics instead of applied. 🙂

The death of a dream

I chuckled when I posted on social media: it has struck me that I had been lifting weights for 42 years (starting in the 8’th grade and being consistent). I was a bit surprised that, after 42 years of weight lifting, that I am so weak! (*)

So, I found at photo of the gym I used. During that period of my life, I just “knew” that I was going to be a professional football player. So I ran, lifted weights and….well…still got run over by those who were destined to play football at the division I level. I simply do not have an athlete’s body…but I really didn’t come to grips with that until I was a senior in high school.

That was a downer for me. The dream died. I still remember getting mail from the football program at the school I ended up attending…it was a form to order tickets. 😦

The point: though I was consistently considerably slower and weaker than those destined to play at the next level, I stayed in denial. If only I ran more sprints, lifted more weights, etc.

What does that have to do with today?

I just gave back my first set of calculus exams. With every group comes a few students who…well, they are going to be an engineer (in some cases, their parents think so). But, try as they might, calculating: is tough for them. is all but impossible for them. Yes, I am talking about the ones who attend classes, study and come to office hours.

It is like me with my 40 yard dash: I did sprints, I ran hills, but when I tried, the stopwatch still said 5.9. Yes, I was that slow; much slower than a typical college lineman.

The reality is that one’s dreams are often out of reach, and sometimes, students find that out in their first college level calculus class.

(*)disclaimer: I am 55 years old, weigh about 183 pounds and regularly do 5 sets of 10 pull ups, and 3 reps with 180 on the bench press; my lifetime best is 310 (when I weighed 230 lbs), which I can’t even take off of the racks now.

How to succeed at calculus, and why it is worth it!

This post is intended to help the student who is willing to put time and effort into succeeding in a college calculus class.

Part One: How to Study

The first thing to remember is that most students will have to study outside of class in order to learn the material. There are those who pick things up right away, but these students tend to be the rare exception.

Think of it this way: suppose you want to learn to play the piano. A teacher can help show you how to play it and provide a practice schedule. But you won’t be any good if you don’t practice.

Suppose you want to run a marathon. A coach can help you with running form, provide workout schedules and provide feedback. But if you don’t run those workouts, you won’t build up the necessary speed and endurance for success.

The same principle applies for college mathematics classes; you really learn the material when you study it and do the homework exercises.

Here are some specific tips on how to study:

1. It is optimal if you can spend a few minutes scanning the text for the upcoming lesson. If you do this, you’ll be alert for the new concepts as they are presented and the concepts might sink in quicker.

2. There is some research that indicates:
a. It is better to have several shorter study sessions rather than one long one and
b. There is an optimal time delay between study sessions and the associated lecture.

Look at it this way: if you wait too long after the lesson to study it, you would have forgotten much of what was presented. If you study right away, then you really have, in essence, a longer class room session. It is probably best to hit the material right when the initial memory starts to fade; this time interval will vary from individual to individual. For more on this and for more on learning for long term recall, see this article.

3. Learn the basic derivative formulas inside and out; that is, know what the derivatives of functions like are on sight; you shouldn’t have to think about them. The same goes for the basic trig identities such as and

Why is this? The reason is that much of calculus (though not all!) boils down to pattern recognition.

For example, suppose you need to calculate:

If you don’t know your differentiation formulas, this problem is all but impossible. On the other hand, if you do know your differentiation formulas, then you’ll immediately recognize the and it’s derivative and you’ll see that this problem is really the very easy problem .

But this all starts with having “automatic” knowledge of the derivative formulas.

Note: this learning is something your professor or TA cannot do for you!

4. Be sure to do some study problems with your notes and your book closed. If you keep flipping to your notes and book to do the homework problems, you won’t be ready for the exams. You have to kick up the training wheels.
Try this; the difference will surprise you. There is also evidence that forcing yourself to recall the material FROM YOUR OWN BRAIN helps you learn the material! Give yourself frequent quizzes on what you are learning.

5. When reviewing for an exam, study the problems in mixed fashion. For example, get some note cards and write problems from the various sections on them (say, some from 3.1, some from 3.2, some from 3.3, and so on), mix the cards, then try the problems. If you just review section by section, you’ll go into each problem knowing what technique to use each time right from the start. Many times, half of the battle is knowing which technique to use with each problem; that is part of the course! Do the problems in mixed order.

If you find yourself whining complaining “I don’t know where to start” it means that you don’t know the material well enough. Remember that a trained monkey can repeat specific actions; you have to be a bit better than that!

6. Read the book, S L O W L Y, with pen and paper nearby. Make sure that you work through the examples in the text and that you understand the reasons for each step.

7. For the “more theoretical” topics, know some specific examples for specific theorems. Here is what I am talking about:

a. Intermediate value theorem: recall that if , then but there is no such that . Why does this not violate the intermediate value theorem?

b. Mean value theorem: note also that there is no such that . Why does this NOT violate the Mean Value Theorem?

c. Series: it is useful to know basic series such as those for . It is also good to know some basic examples such as the geometric series, the divergent harmonic series and the conditionally convergent series .

d. Limit definition of derivative: be able to work a few basic examples of the derivative via the limit definition: and know why the derivative of and do not exist at .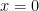

Having some “template” example can help you master a theoretical concept.

Part II: Attitude
Your attitude will be very important.

1. Remember that your effort will be essential! Again, you can’t learn to run a marathon without getting off of the couch and making your muscles sore. Learning mathematics involves some frustration and, yes, at times, some tedium. Learning is fun OVERALL but it isn’t always fun at all times. You will encounter discomfort and unpleasantness at times.

2. Remember that winners look for ways to succeed; losers and whiners look for excuses for failure. You can always find those who will be willing to enable your underachievement. Instead, seek out those who bring out your best.

3. Success is NOT guaranteed; that is what makes success rewarding! Think of how good you’ll feel about yourself if you mastered something that seemed impossible to master at first. And yes, anyone who has achieved anything that is remotely difficult has taken some lumps and bruises along the way. You will NOT be spared these.

Remember that if you duck the calculus challenge, you are, in essence, slamming many doors of opportunity shut right from the get-go.

4. On the other hand, remember that Calculus (the first two semesters anyway) is a Freshman level class; exceptional mathematical talent is not a prerequisite for success. True, calculus is easy for some but that isn’t the point. Most reasonably intelligent people can have success, if they are willing to put forth the proper effort in the proper manner.

Just think of how good it will feel to succeed in an area that isn’t your strong suit!