A tidbit with respect to Laplace transforms and sin(x)/x

I’ve discovered the channel “blackpenredpen” and it is delightful.
It is a nice escape into mathematics that, while far from research level, is “fun” and beyond mere fluff.

And that got me to thinking about . Yes, this can be done by residues

But I’ll look at this with Laplace Transforms.

We know that
But note that the antiderivative of with respect to is That might not seem like much help, but then notice (assuming

So why not:
Now since the left hand side is just a double integral over the first quadrant (an infinite rectangle) the order of integration can be interchanged:

and that is equal to .

Note: is sometimes called the function

\

P-values and precision of language

I read yet another paper proclaiming that it is “now time to do away with p-values.” And yes, I can recommend reading the article.

From my point of view, one of the troubles with p-values is that there is a misunderstanding as to what they actually mean.

So here goes: the p-value is the probability that, given the null hypothesis is true, one obtains an observation as extreme (or greater) than the given observation. That is, if is a random variable with a probability distribution as given by the null hypothesis, and is the observation, .

Example: suppose you assume that a coin is fair (the null hypothesis), and you toss it 100 times and observe 65 heads. It can be shown that . So that is the p-value of that particular experiment. That is, IF the coin really were fair, you’d expect to 65 or more heads .1716 percent of the time.

That seems clear enough, statistically speaking.

But when one gets down to the science, one wants to determine whether there is evidence enough to believe one thing or another thing. So, is this coin biased or did this result happen “just by chance”? And strictly speaking, we don’t really know. For example, it could be that we did a precision scientific measurement on the coin and found it to be fair before doing the above experiment. Or it could be that this was just some coin we came across, or it could be that we were asked to examine this coin because of previous suspicious results. This information matters.

And think of it this way: suppose the above experiment was repeated, say, 100,000 times with a coin known to be fair. Then we’d expect to see the above result about 176 times and ALL of those “positives” would be “due to chance”.

Upshot: when it comes to scientific experiments, we still need replication.

A popular video and covering spaces…

Think back to how you introduced the sine and cosine functions on the real line. Ok, you didn’t do it quite this way, but what you did, in effect, is to define and and then use “elementary trigonometry” to relate the “angle” to the arc length subtended on the circle . One notes that the map defined by has period

Note: the direction “to the right” on the real line is taken to be “counterclockwise” on the circle (red arrows).

Skip if you haven’t had a topology class
The top line is known as the “universal covering space” for the circle. The reason for the terminology has to do with topology. Depending on how long ago you had your topology course, you might remember that the fundamental group of the real line is trivial and the associated group of deck transformations is infinite cyclic (generated by the map ). One then shows that the fundamental group of the circle is the quotient of the group of deck transformations with the fundamental group of the real line; hence the fundamental group of the circle is infinite cylic.

Resume if you haven’t had a topology class

Notice the following: if one, say, “takes a walk” along the line in the direction of the red arrow, the action of the “covering mapping” is to take the same walk in the counter clockwise direction of the circle. That is, the covering action does the following: a walk on the line in the direction of points corresponds to a walk on the circle . That is, walking from to corresponds to a complete lap of the circle.

(that is, on the real line, )

Now note the following: for BOTH the line and the circle, the direction is well defined. “To the right” on the real line” is “counter clockwise” on the circle.

However: on the real line, it makes perfect sense to say that is “before” which is “before” which is “before” and so on; this is merely:

. This is order is valid no matter where one starts on the line.

However, this “universal ordering” makes no sense on the circle, UNLESS one specifies a start point. True, one moves from 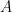to to 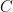and back to 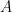again..but if one started at and started to walk, it would appear that 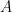came AFTER and not before.

So what?

A friend posted this to her facebook wall:

This quirky animation from CraveFX starts off innocently enough, a janitorial worker mops up a leaky refrigerator and then picks up a coin on the ground. It’s not until you see what causes the refrigerator to leak and why the coin is on the ground that you realize that you’re watching an intricate moving puzzle piece before your eyes. The characters are stuck in an infinite loop caused by another character in their own infinite loop. It’s chaotic and great and hard to keep up with.

The video is below. Now the question: “what action occurred before what other action”? and the answer is “it depends on when you started watching”. The direction of time corresponds to the red arrows in the above diagrams; THAT is well defined. Why? The reason is the Second Law of Thermodynamics; spills do NOT reverse themselves, hence the direction is set in stone, so to speak. But as far as order, it depends ON WHEN THE VIEWER STARTED WATCHING.

Anyway, this video reminded me of covering spaces.

J. H. Conway, Terry Tao and avoiding work

The mainstream media recently had some excellent articles on two mathematical giants:

John Conway and Terrance Tao. I’ve never met Terry Tao though I do read (or try to follow) his blog.

I did meet John Conway when he visited the University of Texas. He is a friend of my dissertation advisor and gave some talks on knot diagram colorings.

I had a private conversation with him at a party, and he gave me some ideas which resulted in three papers for me! Here is one of them.

Yes, I am avoiding studying a book on the theory of interest; I am teaching that course this fall and need to get ahead of the game.

Unfortunately, when I don’t teach, my use of time becomes undisciplined.

Dinette set on calculus…

Note: if you haven’t followed Julie Larson’s comic strip Dinette Set, the characters featured in it are not, well, the world’s most intellectually minded characters (with the exception of Patty). 🙂

Ironically, I see such attitudes displayed by people…posting their thoughts on the internet via a computer or smart phone. The irony doesn’t even occur to them.

Differential equations mentioned in National Review

(hat tip: Vox)

The National Review excerpt:

[…]One part insecure hipsterism, one part unwarranted condescension, the two defining characteristics of self-professed nerds are (a) the belief that one can discover all of the secrets of human experience through differential equations and (b) the unlovely tendency to presume themselves to be smarter than everybody else in the world. Prominent examples include […]

(emphasis mine).

Oh noes! I love differential equations! 🙂

Yeah, I am just having fun with the quote; I couldn’t resist mentioning an article in the popular press that mentions differential equations. I am not sure that I’ll teach the chapter on “all the secrets of human experience” in my upcoming differential equations class though.

Math before symbolic notation had been invented…

There is a tendency to take modern symbolic notation for granted. Here is an excellent “popular” article about the invention of algebraic notation.

This might be something nice to share with calculus students.

Blogging will be very light as I work on revisions for a paper.

Projections, regressions and Anscombe’s quartet…

Data and its role in journalism is a hot topic among some of the bloggers that I regularly follow. See: Nate Silver on what he hopes to accomplish with his new website, and Paul Krugman’s caveats on this project. The debate is, as I see it, about the role of data and the role of having expertise in a subject when it comes to providing the public with an accurate picture of what is going on.

Then I saw this meme on a Facebook page:

These two things (the discussion and meme) lead me to make this post.

First the meme: I thought of this meme as a way to explain volume integration by “cross sections”. 🙂 But for this post, I’ll focus on this meme showing an example of a “projection map” in mathematics. I can even provide some equations: imagine the following set in described as follows: Now the projection map to the plane is given by and the image set is which is a disk (in the yellow).

The projection onto the plane is given by and the image is which is a rectangle (in the blue).

The issue raised by this meme is that neither projection, in and of itself, determines the set . In fact, both of these projections, taken together, do not determine the object. For example: the “hollow can” in the shape of our would have the same projection; there are literally an uncountable. Example: imagine a rectangle in the shape of the blue projection joined to one end disk parallel to the yellow plane.

Of course, one can put some restrictions on candidates for (the pre image of both projections taken together); say one might want to be a manifold of either 2 or 3 dimensions, or some other criteria. But THAT would be adding more information to the mix and thereby, in a sense, providing yet another projection map.

Projections, by design, lose information.

In statistics, a statistic, by definition, is a type of projection. Consider, for example, linear regression. I discussed linear regressions and using “fake data” to teach linear regression here. But the linear regression process inputs data points and produces numbers including the mean and standard deviations of the values as well as the correlation coefficient and the regression coefficients.

But one loses information in the process. A good demonstration of this comes from Anscombe’s quartet: one has 4 very different data set producing identical regression coefficients (and yes, correlation coefficients, confidence intervals, etc). Here are the plots of the data:

And here is the data:

The Wikipedia article I quoted is pretty good; they even provide a link to a paper that gives an algorithm to generate different data sets with the same regression values (and yes, the paper defines what is meant by “different”).

Moral: when one crunches data, one has to be aware of the loss of information that is involved.

Why don’t you people use NUMBERS instead of letters?

Yes, some people have asked me this: “why all of the letters? Why don’t you use NUMBERS?”.

If I am in a patient mood I might say something like: “ok, suppose you want to be able to program a computer to compute a tax on an order? Well, you’d need the item ordered, the price of the item ordered, how many of each item ordered and the applicable tax, right?

Well, there is a “slot” in the order form for each of those, and the “letters” we use stand for such slots.”

Usually, these questions come from those who haven’t had the benefit of an education.

I expect better from our political leaders, and from time to time I am disappointed in them:

Ignoring pleas from business leaders, the Senate Education Committee voted 6-3 along party lines Thursday to bar Arizona from implementing the Common Core standards the state adopted four years ago.

Sen. Al Melvin, R-Tucson, who championed SB 1310, said he believes the concept of some nationally recognized standards started out as a “pretty admirable pursuit by the private sector and governors.”

“It got hijacked by Washington, by the federal government,” said Melvin, a candidate for governor, and “as a conservative Reagan Republican I’m suspect about the U.S. Department of Education in general, but also any standards that are coming out of that department.”

Melvin’s comments led Sen. David Bradley, D-Tucson, to ask him whether he’s actually read the Common Core standards, which have been adopted by 45 states.

“I’ve been exposed to them,” Melvin responded.

Pressed by Bradley for specifics, Melvin said he understands “some of the reading material is borderline pornographic.” And he said the program uses “fuzzy math,” substituting letters for numbers in some examples.

No, this isn’t satire. I wish that it were.

Note while there ARE legitimate criticisms of Common Core; using “letters for numbers” isn’t one of them.

Note: the above link was brought to my attention by someone on Facebook.

The Daily Jumble: Math version (Foxtrot)

GROAN.

This isn’t the first for Foxtrot though: