Teaching double integrals: why you should *always* sketch the region

The problem (from Larson’s Calculus, an Applied Approach, 10’th edition, Section 7.8, no. 18 in my paperback edition, no. 17 in the e-book edition) does not seem that unusual at a very quick glance:

if you have a hard time reading the image. AND, *if* you just blindly do the formal calculations:

which is what the text has as “the answer.”

But come on. We took a function that was negative in the first quadrant, integrated it entirely in the first quadrant (in standard order) and ended up with a positive number??? I don’t think so!

Indeed, if we perform which is far more believable.

So, we KNOW something is wrong. Now let’s attempt to sketch the region first:

Oops! Note: if we just used the quarter circle boundary we obtain

The 3-dimensional situation: we are limited by the graph of the function, the cylinder and the planes ; the plane is outside of this cylinder. (from here: the red is the graph of

Now think about what the “formal calculation” really calculated and wonder if it was just a coincidence that we got the absolute value of the integral taken over the rectangle

Integrals of functions with nice inverses

This idea started as a bit of a joke:

https://twitter.com/lightediand/status/1437181306526371845

Of course, for readers of this blog: easy-peasy. so the integral is transformed into and so we’ve entered the realm of rational functions. Ok, ok, there is some work to do.

But for now, notice what is really doing on: we have a function under the radical that has an inverse function (IF we are careful about domains) and said inverse function has a derivative which is a rational function

More shortly: let be such that then:

gets transformed: and then and the integral becomes which is a rational function integral.

Yes, yes, we need to mind domains.

Vaccine efficacy wrt Hospitalization

I made a short video; no, I did NOT have “risk factor”/”age group” breakdown, but the overall point is that vaccines, while outstanding, are NOT a suit of perfect armor.

Upshot: I used this local data:

The vaccination rate of the area is slightly under 50 percent; about 80 percent for the 65 and up group. But this data doesn’t break it down among age groups so..again, this is “back of the envelope”:

or about 77 percent efficacy with respect to hospitalization, and or 93.75 percent with respect to ending up in the ICU.

Again, the efficacy is probably better than that because of the lack of risk factor correction.

Note: the p-value for the statistical test of vaccines have no effect on hospitalization” vs. “effect” is

The video:

Logarithmic differentiation: do we not care about domains anymore?

The introduction is for a student who might not have seen logarithmic differentiation before: (and yes, this technique is extensively used..for example it is used in the “maximum likelihood function” calculation frequently encountered in statistics)

Suppose you are given, say, and you are told to calculate the derivative?

Calculus texts often offer the technique of logarithmic differentiation: write
Now differentiate both sides:

Now multiply both sides by to obtain

\

And this is correct…sort of. Why I say sort of: what happens, at say, ? The derivative certainly exists there but what about that second factor? Yes, the sin(x) gets cancelled out by the first factor, but AS WRITTEN, there is an oh-so-subtle problem with domains.

You can only substitute only after simplifying ..which one might see as a limit process.

But let’s stop and take a closer look at the whole process: we started with and then took the log of both sides. Where is the log defined? And when does ? You got it: this only works when .

So, on the face of it, is justified only when each .

Why can we get away with ignoring all of this, at least in this case?

Well, here is why:

1. If is a differentiable function then
Yes, this is covered in the derivation of material but here goes: write

Now if we get as usual. If then and so in either case:

as required.

THAT is the workaround for calculating where : just calculate . noting that

Yay! We are almost done! But, what about the cases where at least some of the factors are zero at, say ?

Here, we have to bite the bullet and admit that we cannot take the log of the product where any of the factors have a zero, at that point. But this is what we can prove:

Given is a product of differentiable functions and then

This works out to what we want by cancellation of factors.

Here is one way to proceed with the proof:

1. Suppose are differentiable and . Then and
2. Now suppose are differentiable and . Then and
3.Now apply the above to is a product of differentiable functions and
If then by inductive application of 1.

If then let as in 2. Then by 2, we have Now this quantity is zero unless and . But in this case note that

So there it is. Yes, it works ..with appropriate precautions.

This always bothered me about partial fractions…

Let’s look at an “easy” starting example: write
We know how that goes: multiply both sides by to get and then since this must be true for ALL , substitute to get and then substitute to get . Easy-peasy.

BUT…why CAN you do such a substitution since the original domain excludes ?? (and no, I don’t want to hear about residues and “poles of order 1”; this is calculus 2. )

Lets start with with the restricted domain, say
Now multiply both sides by and note that, with the restricted domain we have:

But both sides are equal on the domain and the limit on the left hand side is So the right hand side has a limit which exists and is equal to . So the result follows..and this works for the calculation for B as well.

Yes, no engineer will care about this. But THIS is the reason we can substitute the non-domain points.

As an aside: if you are trying to solve something like one can do the denominator clearing, and, as appropriate substitute and compare real and imaginary parts ..and yes, now you can use poles and residues.

Elementary Algebra Exercises

I saw this meme floating around:

So:

1. Assuming that are real numbers, find all for which each relation is true, OR show why it is impossible.

2. Where appropriate, repeat exercise 1 but for, say, a field or ring.

Over-scheduling of senior faculty and lower division courses: how important is course prep?

It seems as if the time faculty is expected to spend on administrative tasks is growing exponentially. In our case: we’ve had some administrative upheaval with the new people coming in to “clean things up”, thereby launching new task forces, creating more committees, etc. And this is a time suck; often more senior faculty more or less go through the motions when it comes to course preparation for the elementary courses (say: the calculus sequence, or elementary differential equations).

And so:

1. Does this harm the course quality and if so..
2. Is there any effect on the students?

I should first explain why I am thinking about this; I’ll give some specific examples from my department.

1. Some time ago, a faculty member gave a seminar in which he gave an “elementary” proof of why is non-elementary. Ok, this proof took 40-50 minutes to get through. But at the end, the professor giving the seminar exclaimed: “isn’t this lovely?” at which, another senior member (one who didn’t have a Ph. D. had had been around since the 1960’s) asked “why are you happy that yet again, we haven’t had success?” The fact that a proof that could not be expressed in terms of the usual functions by the standard field operations had been given; the whole point had eluded him. And remember, this person was in our calculus teaching line up.

2. Another time, in a less formal setting, I had mentioned that I had given a brief mention to my class that one could compute and improper integral (over the real line) of an unbounded function that that a function could have a Laplace transform. A junior faculty member who had just taught differential equations tried to inform me that only functions of exponential order could have a Laplace transform; I replied that, while many texts restricted Laplace transforms to such functions, that was not mathematically necessary (though it is a reasonable restriction for an applied first course). (briefly: imagine a function whose graph consisted of a spike of height at integer points over an interval of width and was zero elsewhere.

3. In still another case, I was talking about errors in answer keys and how, when I taught courses that I wasn’t qualified to teach (e. g. actuarial science course), it was tough for me to confidently determine when the answer key was wrong. A senior, still active research faculty member said that he found errors in an answer key..that in some cases..the interval of absolute convergence for some power series was given as a closed interval.

I was a bit taken aback; I gently reminded him that was such a series.

I know what he was confused by; there is a theorem that says that if converges (either conditionally or absolutely) for some then the series converges absolutely for all 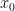where The proof isn’t hard; note that convergence of means eventually, for some positive then compare the “tail end” of the series: use and then and compare to a convergent geometric series. Mind you, he was teaching series at the time..and yes, is a senior, research active faculty member with years and years of experience; he mentored me so many years ago.

4. Also…one time, a sharp young faculty member asked around “are there any real functions that are differentiable exactly at one point? (yes: try if is rational, if is irrational.

5. And yes, one time I had forgotten that a function could be differentiable but not be (try: at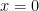

What is the point of all of this? Even smart, active mathematicians forget stuff if they haven’t reviewed it in a while…even elementary stuff. We need time to review our courses! But…does this actually affect the students? I am almost sure that at non-elite universities such as ours, the answer is “probably not in any way that can be measured.”

Think about it. Imagine the following statements in a differential equations course:

1. “Laplace transforms exist only for functions of exponential order (false)”.
2. “We will restrict our study of Laplace transforms to functions of exponential order.”
3. “We will restrict our study of Laplace transforms to functions of exponential order but this is not mathematically necessary.”

Would students really recognize the difference between these three statements?

Yes, making these statements, with confidence, requires quite a bit of difference in preparation time. And our deans and administrators might not see any value to allowing for such preparation time as it doesn’t show up in measures of performance.

Proving a differentiation formula for f(x) = x ^(p/q) with algebra

Yes, I know that the proper way to do this is to prove the derivative formula for and then use, say, the implicit function theorem or perhaps the chain rule.

But an early question asked students to use the difference quotient method to find the derivative function (ok, the “gradient”) for And yes, one way to do this is to simplify the difference quotient by factoring from both the numerator and the denominator of the difference quotient. But this is rather ad-hoc, I think.

So what would one do with, say, where are positive integers?

One way: look at the difference quotient: and do the following (before attempting a limit, of course): let at which our difference quotient becomes:

Now it is clear that is a common factor..but HOW it factors is essential.

So let’s look at a little bit of elementary algebra: one can show:

(hint: very much like the geometric sum proof).

Using this:

Now as

we have (for the purposes of substitution) so we end up with:

(the number of terms is easy to count).

Now back substitute to obtain which, of course, is the familiar formula.

Note that this algebraic identity could have been used for the old case to begin with.

Elementary Math in the news: elections

Ok, mostly I am trying to avoid writing up the painful details of a proposed mathematics paper.
But I do follow elections relatively closely. In the California Democratic primary, CNN called the election for Hillary Clinton late on June 7; at the time she lead Bernie Sanders 1,940,588-1,502,043, which is a margin of 438,537 votes. Percentage wise, the lead was 55.8-43.2, or 12.6 percentage points.

But due to mail in balloting and provisional ballot counting, there were still many votes to count. As of this morning, the totals were:

2,360,266-1,887,178 for a numerical lead of 473,088 votes. Percentage wise, the lead was 55.1-44.0, or 11.1 percentage points.

So, the lead grew numerically, but shrunk percentage wise.

“Big deal”, you say? Well, from reading social media, it is not obvious (to some) how a lead can grow numerically but shrink as a percentage.

Conceptually, it is pretty easy to explain: suppose one has an election involving 1100 voters who MUST choose between candidates. Say the first 100 votes that are counted happened to come from a strongly pro-Hillary group, and the tally after 100 was 90 Hillary, 10 Bernie. Then suppose the next 1000 was closer, say 550 for Hillary and 450 for Bernie. Then the lead grew by 100 votes (80 to 180) but the percentage lead shrunk from 80 percentage points to a 16.36 percentage point lead (58.18 to 41.82 percent). And it is easy to see that if the rest of the vote was really 55 percent Hillary, her percent of the vote would asymptotically shrink to close to 55 percent as the number of votes counted went up.

So, how might one have students model it? Let be increasing functions of which represent the number of votes for Hillary and Bernie as a function of time. Assume no mistakes, hence can be assumed to be increasing functions. So we want a case there is an increasing function but decreases with time.

Without calculus: rewrite and note that decreases as increases; that is, as outgrows . But must continue to outgrow . That is, the new ballots must still include more Hillary Bernie ballots, but the ratio of Bernie ballots to Hillary ballots must be going down.

If we use some calculus, we see that must exceed but to make decrease, use the quotient rule plus a tiny bit of algebra to conclude that must be negative, or that . That is, the Bernie ballots must be growing at a higher percentage rate than the Hillary ballots are.

None of this is surprising, but it might let the students get a feel of what derivatives are and what proportional change means.

Multi leaf polar graphs and total area…

I saw polar coordinate calculus for the first time in 1977. I’ve taught calculus as a TA and as a professor since 1987. And yet, I’ve never thought of this simple little fact.

Consider . Now it is well know that the area formula (area enclosed by a polar graph, assuming no “doubling”, self intersections, etc.) is

Now the leaved roses have the following types of graphs: leaves if is odd, and leaves if is even (in the odd case, the graph doubles itself).

So here is the question: how much total area is covered by the graph (all the leaves put together, do NOT count “overlapping”)?

Well, for an integer, the answer is: if is odd, and if is even! That’s it! Want to know why?

Do the integral: if is odd, our total area is . If is even, we have the same integral but the outside coefficient is which is the only difference. Aside from parity, the number of leaves does not matter as to the total area!

Now the fun starts when one considers a fractional multiple of and I might ponder that some.